Class 11: Physics Chapter 5: Laws of Motion

 Laws of Motion 

LAWS OF MOTION

ARISTOTLE’S FALLACY

• Aristotelian law of motion states: An external force is required to keep a body in motion.
• Galileo disproved this by experiments using inclined planes.

GALILEO’S EXPERIMENTS

Using a single inclined plane

• Galileo studied the motion of objects on an inclined plane.
• Objects moving

1. down an inclined plane, get accelerated,
2. up an inclined plane, retarded,
3. on a horizontal plane, is an intermediate situation.

• Galileo concluded that an object moving on a frictionless horizontal plane should move with constant velocity.

Using Double inclined plane

 

• A ball released from rest on one of the planes rolls down and climbs up the other.
• When friction is absent, the final height of the ball is the same as its initial height.
• If the slope of the second plane is decreased and the experiment repeated, the ball will still reach the same height, but it will travel a longer distance.
• When the slope of the second plane is zero (i.e., is a horizontal) the ball travels an infinite distance.
• If there were no friction, the ball would continue to move with a constant velocity on the horizontal plane.
• Thus, according to Galileo, the state of rest and the state of uniform linear motion (motion with constant velocity) are equivalent.
• If the net external force is zero, a body at rest continues to remain at rest and a body in motion continues to move with a uniform velocity.

NEWTON’S LAWS OF MOTION

• Starting from Galileo’s ideas, Newton formed three laws of motion.

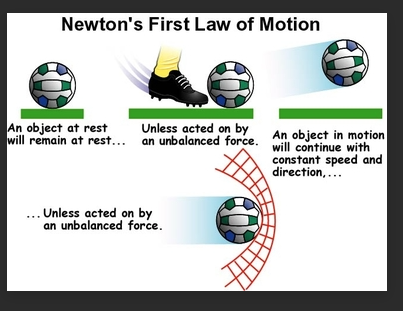
NEWTON’S FIRST LAW OF MOTION – THE LAW OF INERTIA

• Everybody continues to be in its state of rest or of uniform motion in a straight line unless and until compelled by some external force to act otherwise.

INERTIA

• The inability of a body to change its state of rest or uniform motion along a straight line.
• Inertia means resistance to change.

Inertia of rest (static inertia)

• The property of an object by virtue of which it remains in its state of rest.
• Examples:
  1. a person standing in a bus tends to fall backward when the bus starts suddenly
  2. Fruits fall down due to inertia of rest when the branches of a tree are shaken
  3. Dust particles on a carpet fall if we beat the carpet with a stick
  4. With a quick pull, a table cloth can be removed from a dining table without disturbing dishes on it due to the Inertia of rest.

Inertia of motion (kinetic inertia)

• The property by which it remains in state of motion

1. A person in running bus , falls forward the bus when stops suddenly.
2. Athlete taking a short run before a jump.

Forces on a book at rest on a horizontal surface

The two forces are

i) the force due to gravity (i.e., its weight W) acting downward

ii) the upward force on the book by the table, the normal force R.

• Since the book is rest the net force on the book must be zero.

Forces on a car

• When the car is stationary, there is no net force acting on it.
• During pick-up, it accelerates. It is the frictional force that accelerates the car as a whole.
• When the car moves with constant velocity, there is no net external force.
• Friction on the front wheels opposes the spinning, so friction must point in the forward direction.

MOMENTUM ( P )

• Momentum is the product of its mass and velocity

Momentum is a vector quantity

Some Situations relating momentum and applied force 

Situation -1 

• A much greater force is needed to push the truck than the car to bring them to the same speed in same time.
• A greater opposing force is needed to stop a heavy body than a light body in the same time, if they are moving with the same speed
• If two stones, one light and the other heavy, are dropped from the top of a building, a person on the ground will find it easier to catch the light stone than the heavy stone.

Reason

• In these cases, change in momentum is greater for a heavy body.
• External force required is proportional to change in momentum for the given time.

Situation -2

1. A bullet fired by a gun can easily pierce human tissue before it stops, resulting in casualty.
2. The same bullet fired with moderate speed will not cause much damage

Reason

• Velocity is high for a bullet from a gun – the change in momentum is high
• External force required to stop the bullet is proportional to change in momentum for a given time.

Situation -3

i) A seasoned cricketer catches a cricket ball coming in with great speed far more easily than a novice, who can hurt his hands in the act

Reason

• External force depends on the time in which the momentum change is brought about.
• The change in momentum brought about in a shorter time needs greater applied force and vice versa.

Situation -4

• Suppose a stone is rotated with uniform speed in a horizontal plane by means of a string, the magnitude of momentum is fixed, but its direction changes
• The force needed to change in momentum is provided by our hand through the string.
• Our hand needs to exert a greater force if the stone is rotated at greater speed or in a circle of smaller radius, or both.

Reason

• External force is proportional to change in momentum.

 

NEWTON’S SECOND LAW OF MOTION

• The rate of change of momentum of a body is directly proportional to the applied force and takes place in the direction in which the force acts.

• This law is applicable to both single particle and a system of particles.

Definition of Newton

• One newton is that force, which causes an acceleration of 1m/s2,

 to a mass of 1 kg.

 

Newton’s First Law from Second Law

• 
  

Newton’s second law in vector component form

 

The three rectangular components of Force can be represented as follows.

PROBLEM

• A bullet of mass 0.04 kg moving with a speed of 90 m s-1 enters a heavy wooden block and is stopped after a distance of 60 cm. What is the average resistive force exerted by the block on the bullet?

Solution

• Given m=0.04kg, v0 = 90 m/s , x= 0.6m, v=0
• The acceleration of the bullet is given by

Impulse

• The product of force and time.

 = Change in Momentum

• Unit of impulse is newton-second (Ns).

Impulsive force

• A large force acting for a short time to produce a finite change in momentum.
• Examples are force when a ball hits on a wall, force exerted by a bat on a ball, force on a nail by a hammer etc.

PROBLEM

• A batsman hits back a ball straight in the direction of the bowler without changing its initial speed of 12 m s–1. If the mass of the ball is 0.15 kg, determine the impulse imparted to the ball. (Assume linear motion of the ball

Solution

Impulse = Change in momentum

NEWTON’S THIRD LAW OF MOTION

• To every action, there is always an equal and opposite reaction.
• Forces always occur in pairs. Force on a body A by B is equal and opposite to the force on the body B by A.
• Action and reaction force occurs simultaneously.
• Action and reaction forces act on different bodies, not on the same body – they do not cancel each other.

Examples

• When a man walks on earth he exerts a force in the backward direction- Action The earth exerts an equal reaction on man in the forward direction. As a result, he moves in forward direction.
• When a bird flies it exerts a force on the air by its wings.

The air exerts a reaction force on the wings in the opposite direction. As a result, the bird flies.

• When a bullet is fired from a gun , the force exerted on the bullet is action. The bullet exerts a reaction force on the gun in the opposite direction- recoil of gun
• In a rocket the burnt gas at a very high-pressure escapes through the nozzle with a tremendous force.

This escaping gas exerts a reaction force on the rocket in the opposite direction. Thus, rocket moves forward

PROBLEM

• Two identical billiard balls strike a rigid wall with the same speed but at different angles, and get reflected without any change in speed as shown in fig. What is

1. The direction of the force on the wall due to each ball?
2. the ratio of the magnitudes of impulses imparted to the balls by the wall?

Solution

i) To find the direction of force, impulse (change in momentum) of the ball is

calculated.

Case (a)

• Therefore x – component of impulse = – 2mu
• y – component of impulse = 0
• Impulse and force are in the same direction
• Thus, the force on the ball due to the wall is normal to the wall, along the negative X-direction.
• From Newton’s Third law, the force on the wall due to the ball is normal to the wall, along the positive x-direction.

Case (b)

• Thus

• Therefore, the force on the wall due to the ball is normal to the wall, along the positive X-direction.

ii) The ratio of the magnitudes of the impulses imparted to the balls is

THE LAW OF CONSERVATION OF MOMENTUM

• The total momentum of an isolated system ( a system with no external force ) of interacting particles is conserved.
• From Newton’s second law  

• Therefore, when F=0, initial momentum = final momentum.

 

Applications of conservation of momentum:

 Recoil of a gun

• Velocity of a bullet- muzzle velocity
• Movement of gun backward, when a bullet is fired- recoil of gun
• According to conservation of momentum momentum before firing = momentum after firing.

• Thus

Where m- mass of bullet, u- velocity of bullet, M- mass of gun, V- recoil velocity of gun

• Therefore

• The negative sign shows that velocity of gun is opposite to that of bullet
• Recoil velocity is very small (since M > m)

 

Rocket propulsion

• When a rocket is fired, fuel is burnt in the combustion chamber.
• The hot gas at very high-pressure escapes through the nozzle with a very high velocity
• The escaping gas has a very high momentum
• In order to conserve momentum, the rocket moves in the forward direction.

 

EQUILIBRIUM OF A PARTICLE

• Equilibrium of a particle in mechanics refers to the situation when the net external force on the particle is zero.
• The forces acting at a point are called concurrent forces
• If two forces F1 and F2, act on a particle, equilibrium requires

• Equilibrium under three concurrent forces F1, F2 and F3 requires that

COMMON FORCES IN MECHANICS

i) Gravitational force –

• Every object on the earth experiences the force of gravity due to the earth.

ii) Contact forces

• A contact force on an object arises due to contact with some other object: solid or fluid.
• When bodies are in contact there are mutual contact forces (for each pair of bodies) satisfying the third law.
• The component of contact force normal to the surfaces in contact is called normal reaction.
• The component parallel to the surfaces in contact is called friction.

iii) Force due to spring

• When a spring is compressed or extended by an external force, a restoring force is generated.
• This force is usually proportional to the compression or elongation (for small displacements).
• The spring force F is written as F = – k x where x is the displacement and k is the force constant.
• The negative sign denotes that the force is opposite to the displacement from the unstretched state.

iv) Tension in a string ( T )

• The restoring force in a string is called tension.
• The direction is always away from the body

The different contact forces of mechanics mentioned above fundamentally arise from electrical forces.

MOTION OF CONNECTED BODIES

Body moving on a horizontal frictionless table

Motion in a frictionless pulley

• And

Motion in a lift

a) Lift at rest or moving with constant velocity upward or downward

• Here acceleration a=0
• The net force is

• Thus apparent weight = Actual weight

b) Lift moves up with acceleration a

• The net force

• Apparent weight > Actual weight

c) Lift moves down with acceleration a

• The net force

• Apparent weight < Actual weight

d) Lift falls down freely

FRICTION

• Friction is the force which opposes the relative motion between two surfaces in contact.
• It acts tangential to the surface of contact.
• Arises due to

1. adhesive force between surfaces
2. irregularities of plane surface

• There are two types

i) Static friction

ii) Kinetic friction

Alternative Script

Friction is of three types: 

1. Static Friction 

It is an opposing force which comes into play when one body tends to move over the surface of the other body but actual motion is not taking place. Static friction is a self adjusting force which increases as the applied force is increased, 

2. Limiting Friction 

 It is the maximum value of static friction when body is at the verge of starting motion. 

Limiting friction (fs) = μsR 

where μs , = coefficient of limiting friction and R = normal reaction.

 Limiting friction does not depend on area of contact surfaces but depends on their nature, i.e., smoothness or roughness. 

If angle of friction is θ, then 

coefficient of limiting friction μs = tan θ 

3. Kinetic Friction 

If the body begins to slide on the surface, the magnitude of the frictional force rapidly decreases to a constant value fk, kinetic friction. 

Kinetic friction, fk = μk N 

where μ k = coefficient of kinetic friction and N = normal force. 

 Kinetic friction, fk = μk N 

where μ k = coefficient of kinetic friction and N = normal force. 

Kinetic friction is of two types: 

(a) Sliding friction 

(b) Rolling friction 

As, rolling friction < sliding friction, therefore it is easier to roll a body than to slide. 

Kinetic friction (fk) = μk R 

where μk = coefficient of kinetic friction and R = normal reaction. 

Angle of repose or angle of sliding 

It is the minimum angle of inclination of a plane with the horizontal, such that a body placed on it, just begins to slide down. 

If angle of repose is a. and coefficient of limiting friction is μ, 

then μs = tan α 

Static friction

• Friction between two surfaces in contact as long as the bodies is at rest.

• Its value increases from zero to a maximum value called limiting friction (fs ) max.
• Limiting friction is the static frictional force just before sliding.

Laws of Static Friction

• The magnitude of limiting friction is independent of area of the contact between the bodies.
• The limiting friction is proportional to the normal reaction N.

• Thus , value of static friction may be written as

Angle of friction (θ)

• The angle at which the body begin to slide on an inclined plane is called angle of limiting static friction or angle of repose
• The weight of the body can be resolved in to two components.
• Just before sliding

• Dividing the two equations

• Thus, coefficient of static friction is the tangent of the angle of limiting friction.

PROBLEM-1

• Determine the maximum acceleration of the train in which a box lying on its floor will remain stationary, given that the co-efficient of static friction between the box and the train’s floor is 0.15.

Solution

• Since the acceleration of the box is due to the static friction,

PROBLEM-2

• A mass of 4 kg rests on a horizontal plane. The plane is gradually inclined until at an angle = 15° with the horizontal, the mass just begins to slide. What is the coefficient of static friction between the block and the surface?

Solution

Kinetic friction

• Friction experienced by a body when it moves

• Two types:

1. Sliding friction
2. Rolling friction

• Rolling friction < sliding friction < static friction

Laws of kinetic friction

• Kinetic friction does not depend on the nature of the two surfaces in contact.
• Kinetic friction is proportional to the normal reaction.

• μk is the coefficient of kinetic friction
• Coefficient of kinetic friction is less than that of static friction

Rolling friction

• Friction when a body rolls on a surface
• Very small compared to sliding friction-surface area of contact is small
• Advantage of Rolling friction is made use in ball bearings

PROBLEM

What is the acceleration of the block and trolley system shown in the figure, if the coefficient of kinetic friction between the trolley and the surface is 0.04? What is the tension in the string? (Take g = 10 m s-2). Neglect the mass of the string.

 

Solution

FRICTION AS A NECESSARY EVIL

• Friction is considered as a necessary evil, because it has both advantages and disadvantages.

Advantages of friction

• We are able to walk on the ground due to friction
• We can hold an object in hand due to friction
• Meteors burn in air due to friction.

Disadvantages of friction

• When a vehicle moves lot of energy is lost to overcome friction
• Excess heat produced in machines causes wear and tear to parts
• Atmospheric friction is disadvantageous to rockets and satellites

Ways to minimize friction

• Using lubricants like, grease, oil, wax etc.
• Using ball bearings or roll bearings
• Using anti-friction metals or alloys
• Separating the surfaces by an air cushion • Streamlining the body of vehicles
• Polishing the surfaces.

CIRCULAR MOTION

• Acceleration of a body moving in a circle of radius R with uniform speed v is v2/R directed towards the centre.
• According to the second law, the force providing this acceleration is

• This force directed forwards the centre is called the centripetal force.
• For a stone rotated in a circle by a string, the centripetal force is provided by the tension in the string.
• The centripetal force for motion of a planet around the sun is the gravitational force on the planet due to the sun.
• For a car taking a circular turn on a horizontal road, the centripetal force is the force of friction.

Motion of a car on a level road

Three forces act on the car

1. The weight of the car, mg
2. Normal reaction, N
3. Frictional force, f

Maximum speed of the car on a level road

• As there is no acceleration in the vertical direction

• The centripetal force required for the circular motion is provided by the frictional force between road and the car tyres.
• Thus

• Thus, for a given value of μs and R, the maximum speed of circular motion of the car is given by

Motion of a car on a banked road

Banking of roads

• The phenomenon of raising outer edge of the curved road above the inner edge is called banking of roads.
• We can reduce the contribution of friction to the circular motion of the car if the road is banked

Forces on a car in a banked road

Maximum possible speed of a car on a banked road – with friction

• Since there is no acceleration along the vertical direction, the net force along this direction must be zero.
• Thus

• The centripetal force is provided by the horizontal components of N and f.

• Dividing numerator and denominator by cosθ, we get

• Thus, maximum possible speed of a car on a banked road is greater than that on a flat road.

Speed of the car – without friction

• If there is no friction, µs=0,therefore the speed of the car is

• This is called the optimum speed.
• At this speed, frictional force is not needed to provide the necessary centripetal force.
• Driving at this speed on a banked road will cause little wear and tear of the tyres.

PROBLEM-1

• A cyclist speeding at 18 km/h on a level road takes a sharp circular turn of radius 3 m without reducing the speed. The coefficient of static friction between the tyres and the road is 0.1 Will the cyclist slip while taking the turn ?

Solution

PROBLEM -2

• A circular racetrack of radius 300 m is banked at an angle of 15°. If the coefficient of friction between the wheels of a race-car and the road is 0.2, what is the (a) optimum speed of the racecar to avoid wear and tear on its tyres, and (b) maximum permissible speed to avoid slipping ?

Solution

• Given , =150 ,µs =0.2, R=300m, g =9.8m/s2

a) Optimum speed is

Maximum speed is

Revision at a glance

Dynamics is the branch of physics in which we study the motion of a body by taking into consideration the cause i.e., force which produces the motion.
• Force
Force is an external cause in the form of push or pull, which produces or tries to produce motion in a body at rest, or stops/tries to stop a moving body or changes/tries to change the direction of motion of the body.
• The inherent property, with which a body resists any change in its state of motion is called inertia. Heavier the body, the inertia is more and lighter the body, lesser the inertia.
• Law of inertia states that a body has the inability to change its state of rest or uniform motion (i.e., a motion with constant velocity) or direction of motion by itself.
• Newton’s Laws of Motion
Law 1. A body will remain at rest or continue to move with uniform velocity unless an external force is applied to it.
First law of motion is also referred to as the ‘Law of inertia’. It defines inertia, force and inertial frame of reference.
I here is always a need of ‘frame of reference’ to describe and understand the motion of particle, lhc simplest ‘frame of reference’ used are known as the inertial frames.
A frame of referent, e is known as an inertial frame it, within it, all accelerations of any particle are caused by the action of ‘real forces’ on that particle.
When we talk about accelerations produced by ‘fictitious’ or ‘pseudo’ forces, the frame of reference is a non-inertial one.
Law 2. When an external force is applied to a body of constant mass the force produces an acceleration, which is directly proportional to the force and inversely proportional to the mass of the body.


Law 3. “To every action there is equal and opposite reaction force”. When a body A exerts a force on another body B, B exerts an equal and opposite force on A.
• Linear Momentum
The linear momentum of a body is defined as the product of the mass of the body and its velocity.

• Impulse
Forces acting for short duration are called impulsive forces. Impulse is defined as the product of force and the small time interval for which it acts. It is given by

Impulse of a force is a vector quantity and its SI unit is 1 Nm.
— If force of an impulse is changing with time, then the impulse is measured by finding the area bound by force-time graph for that force.
— Impulse of a force for a given time is equal to the total change in momentum of the body during the given time. Thus, we have

• Law of Conservation of Momentum
The total momentum of an isolated system of particles is conserved.
In other words, when no external force is applied to the system, its total momentum remains constant.

• Recoiling of a gun, flight of rockets and jet planes are some simple applications of the law of conservation of linear momentum.

• Concurrent Forces and Equilibrium
“A group of forces which are acting at one point are called concurrent forces.”
Concurrent forces are said to be in equilibrium if there is no change in the position of rest or the state of uniform motion of the body on which these concurrent forces are acting.
For concurrent forces to be in equilibrium, their resultant force must be zero. In case of three concurrent forces acting in a plane, the body will be in equilibrium if these three forces may be completely represented by three sides of a triangle taken in order. If number of concurrent forces is more than three, then these forces must be represented by sides of a closed polygon in order for equilibrium.


• Commonly Used Forces
(i) Weight of a body. It is the force with which earth attracts a body towards its centre. If M is mass of body and g is acceleration due to gravity, weight of the body is Mg in vertically downward direction.
(ii) Normal Force. If two bodies are in contact a contact force arises, if the surface is smooth the direction of force is normal to the plane of contact. We call this force as Normal force.

Example. Let us consider a book resting on the table. It is acted upon by its weight in vertically downward direction and is at rest. It means there is another force acting on the block in opposite direction, which balances its weight. This force is provided by the table and we call it as normal force.
(iii) Tension in string. Suppose a block is hanging from a string. Weight of the block is acting vertically downward but it is not moving, hence its weight is balanced by a force due to string. This force is called ‘Tension in string’. Tension is a force in a stretched string. Its direction is taken along the string and away from the body under consideration.

• Simple Pulley
Consider two bodies of masses m1 and m2 tied at the ends of an in extensible string, which passes over a light and friction less pulley. Let m1 > m2. The heavier body will move downwards and the lighter will move upwards. Let a be the common acceleration of the system of two bodies, which is given by


• Apparent Weight and Actual Weight
— ‘Apparent weight’ of a body is equal to its ‘actual weight’ if the body is either in a state of rest or in a state of uniform motion.
— Apparent weight of a body for vertically upward accelerated motion is given as
Apparent weight =Actual weight + Ma = M (g + a)
— Apparent weight of a body for vertically downward accelerated motion is given as
Apparent weight = Actual weight Ma = M (g – a).
• Friction
The opposition to any relative motion between two surfaces in contact is referred to as friction. It arises because of the ‘inter meshing’ of the surface irregularities of the two surfaces in contact.
• Static and Dynamic (Kinetic) Friction
The frictional forces between two surfaces in contact (i) before and (ii) after a relative motion between them has started, are referred to as static and dynamic friction respectively. Static friction is always a little more than dynamic friction.
The magnitude of kinetic frictional force is also proportional to normal force.

• Limiting Frictional Force
This frictional force acts when body is about to move. This is the maximum frictional force that can exist at the contact surface. We calculate its value using laws of friction.
Laws of Friction:
(i) The magnitude of limiting frictional force is proportional to the normal force at the contact surface.

(ii) The magnitude of limiting frictional force is independent of area of contact between the surfaces.
• Coefficient of Friction
The coefficient of friction (μ) between two surfaces is the ratio of their limiting frictional force to the normal force between them, i.e.,

• Angle of Friction
It is the angle which the resultant of the force of limiting friction F and the normal reaction R makes with the direction of the normal reaction. If θ is the angle of friction, we have

• Angle of Repose
Angle of repose (α) is the angle of an inclined plane with the horizontal at which a body placed over it just begins to slide down without any acceleration. Angle of repose is given by α = tan⁻¹ (μ)

• Motion on a Rough Inclined Plane
Suppose a motion up the plane takes place under the action of pull P acting parallel to the plane.
• Centripetal Force
Centripetal force is the force required to move a body uniformly in a circle. This force acts along the radius and towards the centre of the circle. It is given by

where, v is the linear velocity, r is the radius of circular path and ω is the angular velocity of the body.
• Centrifugal Force
Centrifugal force is a force that arises when a body is moving actually along a circular path, by virtue of tendency of the body to regain its natural straight line path.
The magnitude of centrifugal force is same as that of centripetal force.



• Motion in a Vertical Circle
The motion of a particle in a horizontal circle is different from the motion in vertical circle. In horizontal circle, the motion is not effected by the acceleration due to gravity (g) whereas in the motion of vertical circle, the motion is not effected by the acceleration due to gravity (g) whereas in the motion of vertical circle, the value of ‘g’ plays an important role, the motion in this case does not remain uniform. When the particle move up from its lowest position P, its speed continuously decreases till it reaches the highest point of its circular path. This is due to the work done against the force of gravity. When the particle moves down the circle, its speed would keep on increasing.

Let us consider a particle moving in a circular vertical path of radius V and centre o tide with a string. L be the instantaneous position of the particle such that

Here the following forces act on the particle of mass ‘m’.

(i) Its weight = mg (vertically downwards).
(ii) The tension in the string T along LO.

We can take the horizontal direction at the lowest point ‘p’ as the position of zero gravitational potential energy. Now as per the principle of conservation of energy,

From this relation, we can calculate the tension in the string at the lowest point P, mid-way point and at the highest position of the moving particle.

When the particle completes its motion along the vertical circle, it is referred to as “Looping the Loop” for this the minimum speed at the lowest position must be √5gr.