Class 12- Chapter 1 : Electric Charges and Field

In Electrostatics, we are only concerned about the charge which is at rest, meaning the charge is not moving like the electric current. 

Chapter–1

ELECTRIC CHARGES AND FIELDS

ELECTRIC CHARGE

Charge is something associated with matter due to which it produces and experiences electric and magnetic effects.

There are two types of charges :

• Positive charge

• Negative charge

Positive and negative charges : Positive charge means the deficiency of electrons while negative charge means excess of electrons. In any neutral body the net charge is equal to zero

i.e., the sum of positive charges is equal to the sum of negative charges.

   

Charge is a scalar quantity and its SI unit is coulomb (C).

CONDUCTORS AND INSULATORS

The materials which allow electric charge (or electricity) to flow freely through them are called conductors. Metals are very good conductors of electricity. Silver, Copper and Aluminum are some of the best conductors of electricity. Our skin is also a conductor of electricity. Graphite is the only non-metal conductor of electricity.

All metals, alloys and graphite have 'free electrons', which can move freely throughout the conductor. These free electrons make metals, alloys and graphite good conductors of electricity.

Aqueous solutions of electrolytes are also conductors.

The materials which do not allow electric charge to flow through them are called non-conductors or insulators.

For example, most plastics, rubber, non-metals (except graphite), dry wood, wax, mica, porcelain, dry air etc., are insulators.

Insulators can be charged but do not conduct electric charge. Insulators do not have 'free electrons' that is why insulators do not conduct electricity.

Induced charge can be lesser or equal to inducing charge (but never greater) and its max. value is given by

Q' = – Q (1 – 1/k), where 'Q' is inducing charge and 'K' is the dielectric const. of the material of the uncharged body.

For metals k = ∞ ⇒ Q' = – Q.

METHODS OF CHARGING

1. By friction : By rubbing two suitable bodies, given in a box one is charged by +ve and another by –ve charge in equal amounts.

+ve : Glass rod, Fur, Dry hair, Wool

-ve : Silk, Ebonite rod, Comb, Amber

Note:- Electric charges remain confined only to the rubbed portion of a non-conductor but in case of a conductor, they spread up throughout the conductor.

2. By conduction : Charging a neutral body by touching it with a charged body is called charging by conduction.

It is important to note that when the bodies are charged by conduction, a charged and an uncharged body are brought into contact and then separated, the two bodies may or may not have equal charges.

If the two bodies are identical the charges on the two will be equal.

If the two bodies are not identical, the charges will be different.

The potential of the two bodies will always be the same.

3. By induction : Charging a body without bringing it in contact with a charged body is called charging by induction.

First rearrangement of charge takes place in metal rod B. When the rod B is connected to earth, electrons flow from earth to the rod B thus making it -vely charged

The magnitude of elementary positive or negative charge (electron) is the same and is equal to 1.6 × 10–19 C.

PROPERTIES OF ELECTRIC CHARGE

• Similar charges repel and dissimilar charges attract each other.

In rare situations you may find similar charged bodies attracting each other. Suppose a big positively charged body is placed near a small positively charged body then because of induction, opposite charge produced on the small body makes it attract the other body.

• A charged body attracts light uncharged bodies, due to polarisation of the uncharged body.

Fig : When a positively charged balloon is placed in contact with the wall, an opposite charge is induced with the wall, the balloon stick to the wall due to electrostatic attraction

• Charge is conserved i.e., the charge can neither be created nor be destroyed but it may simply be transferred from one body to the other.

Thus we may say that the total charge in the universe is constant or we may say that charges can be created or destroyed in equal and opposite pair. For example

(Pair-production process)

Positron is an antiparticle of electrons. It has the same mass as that of electrons but equal negative charge.

(Pair-annihilation process)

• Charge is unaffected by motion
  This is also called charge invariance with motion

Mathematically, (q)at rest = (q)in motion

• Quantisation of charge - A charge is an aggregate of a small unit of charges, each unit being known as a fundamental or elementary charge which is equal to e = 1.6 × 10–19 C. This principle states that charge on any body exists as an integral multiple of electronic charge. i.e. q = ne where n is an integer.

According to the concept of quantisation of charges, the charge q cannot go below e. On a macroscopic scale, this is as good as taking the limit q0 → 0.

Quantisation of electric charge is a basic (unexplained) law of nature. It is important to note that there is no analogous law of quantisation of mass.

Recent studies on high energy physics have indicated the presence of graphs with charge 2e/3, e/3. But since these cannot be isolated and are present in groups with total charge, therefore the concept of elementary charge is still valid.

COULOMB’S LAW

The force of attraction or repulsion between two point charges (q1 and q2) at finite separation (r) is directly proportional to the product of charges and inversely proportional to the square of distance between the charges and is directed along the line joining the two charges.

i.e., or

where ε is the permittivity of the medium between the charges.

If ε0 is the permittivity of free space, then relative permittivity of medium or dielectric constant (K), is given by

 

The permittivity of free space

 

and = 9 × 109 Nm2 C–2.

Also in the CGS system of units.

Coulomb’s law may also be expressed as

 

Let F0 be the force between two charges placed in vacuum then

 

Hence 

Therefore we can conclude that the force between two

charges becomes 1/K times when placed in a medium of

dielectric constant K.

The value of K for different media

DIELECTRIC

A dielectric is an insulator. It is of two types -

• Polar dielectric

• Non-polar dielectric

SIGNIFICANCE OF PERMITTIVITY CONSTANT OR DIELECTRIC CONSTANT

Permittivity constant is a measure of the inverse degree of permission of the medium for the charges to interact.

DIELECTRIC STRENGTH

The maximum value of an electric field that can be applied to the dielectric without its electric breakdown is called its dielectric strength.

DIFFERENCE BETWEEN ELECTROSTATIC FORCE AND GRAVITATIONAL FORCE

Note:- Both electric and gravitational forces follow inverse square law.

VECTOR FORM OF COULOMB’S LAW

SUPERPOSITION PRINCIPLE FOR DISCRETE CHARGE DISTRIBUTION: FORCE BETWEEN MULTIPLE CHARGES

The electric force on q1 due to a number of charges placed in air or vacuum is given by

Note:- Coulomb's law is valid if m and if charges are point charges.

FORCE FOR CONTINUOUS CHARGE DISTRIBUTION

A small element having charge dq is considered on the body. The force on the charge q1 is calculated as follows

Now the total force is calculated by integrating under proper limits.

i.e., 

where is a variable unit vector which points from each dq, towards the location of charge q1 (where dq is a small charge element)

TYPES OF CHARGE DISTRIBUTION

• Volume charge distribution : If a charge, Q is uniformly distributed through a volume V, the charge per unit volume ρ (volume charge density) is defined by

; ρ has unit coulomb/m3.

• Surface charge distribution : If a charge Q is uniformly distributed on a surface of area A, the surface charge density , is defined by the following equation

σ has unit coulomb / m2

• Linear charge distribution : If a charge q is uniformly distributed along a line of length λ, the linear charge density λ, is defined by

, λ has unit coulomb/m.

If the charge is non uniformly distributed over a volume, surface, or line we would have to express the charge densities as

where dQ is the amount of charge in a small volume, surface or length element.

In general, when there is a distribution of direct and continuous charge bodies, we should follow the following steps to find force on a charge q due to all the charges :

• Fix the origin of the coordinate system on charge q.

• Draw the forces on q due to the surrounding charges considering one charge at a time.

• Resolve the force in x and y-axis respectively and find and

• The resultant force is and the direction is given byand the direction is given by

CALCULATION OF ELECTRIC FORCE IN SOME SITUATIONS

• Force on one charge due to two other charges

Resultant force on q due to q1 and q2 are obtained by vector addition of individual forces

The direction of F is given by

• Force due to linear charge distribution

Let AB is a long (length ) thin rod with uniform distribution of total charge Q.

We calculate force of these charges i.e. Q on q which is situated at a distance from the edge of rod AB.

Let dQ is a small charge element in rod AB at a distance x from q .

The force on q due to this element will be

where μ is linear charge density i.e., μ = Q / .

so, newton

POINTS TO REMEMBER

1. When the distance between the two charges placed in vacuum or a medium is increased K-times then the force between them decreases K2-times. i.e., if F0 and F be the initial and final forces between them, then

2. When the distance between the two charges placed in vacuum or a medium is decreased K-times then the force between them increases K2-times. i.e., if Fo and F be the initial and final forces then F = K2Fo

3. When a medium of dielectric constant K is placed between the two charges then the force between them decreases by K-times. i.e., if Fo and F be the forces in vacuum and the medium respectively, then

4. When a medium of dielectric constant K between the charges is replaced by another medium of dielectric constant K' then the force decreases or increases by (K/K') times accordingly as K' is greater than K or K' is less than K.

ELECTRIC FIELD

The space around an electric charge, where it exerts a force on another charge is an electric field.

Electric force, like the gravitational force, acts between bodies that are not in contact with each other. To understand these forces, we involve the concept of the Electric  field. When a mass is present somewhere, the properties of space in the vicinity can be considered to be so altered in such a way that another mass brought to this region will experience a force there. The space where alteration is caused by a mass is called its Gravitational field and any other mass is thought of as interacting with the field and not directly with the mass responsible for it.

Similarly an electric charge produces an electric field around it so that it interacts with any other charges present there. One reason it is preferable not to think of two charges as exerting forces upon each other directly is that if one of them is changed in magnitude or position, the consequent change in the forces each experiences does not occur immediately but takes a definite time to be established. This delay cannot be understood on the basis of coulomb law but can be explained by assuming (using field concept) that changes in field travel with a finite speed. (≈ 3 × 108 m / sec).

Electric fields can be represented by field lines or lines of force.

The direction of the field at any point is taken as the direction of the force on a positive charge at the point.

Electric field intensity due to a charge q at any position () from that charge is defined as

where is the force experienced by a small positive test charge q0 due to charge q.

Its SI unit is NC–1. It is a vector quantity.

If there are more charges responsible for the field, then

where are the electric field intensities due to charges q1, q2, q3.....respectively.

ELECTRIC LINES OF FORCE

These are the imaginary lines of force and the tangent at any point on the lines of force gives the direction of the electric field at that point.

PROPERTIES OF ELECTRIC LINES OF FORCE

1. The lines of force diverge out from a positive charge and converge at a negative charge. i.e. the lines of force are always directed from higher to lower potential.

            

2. The electric lines of force contract length wise indicating unlike charges attract each other and expand laterally indicating like charges repel each other.

3. The number of lines that originate from or terminate on a charge is proportional to the magnitude of charge.

i.e.,

4. Two electric lines of force never intersect each other.

5. They begin from positive charge and end on negative charge i.e., they do not make closed loop (while magnetic field lines form closed loop).

6. Where the electric lines of force are

1. close together, the field is strong (see fig.1)

2. far apart, the field is weak (see fig.2)

7. Electric lines of force generate or terminate at charges /surfaces at right angles.

ELECTRIC FIELD FOR CONTINUOUS CHARGE DISTRIBUTION

If the charge distribution is continuous, then the electric field strength at any point may be calculated by dividing the charge into infinitesimal elements. If dq is the small element of charge within the charge distribution, then the electric field at point P at a distance r from charge element dq is

Non conducting sphere (dq is small charge element)

dq = λdl (line charge density)

= σ ds (surface charge density)

= ρdv (volume charge density)

The net field strength due to entire charge distribution is given by

where the integration extends over the entire charge distribution.

Note:- Electric field intensity due to a point charge q, at a distance (r1 + r2) where r1 is the thickness of medium of dielectric constant K1 and r2 is the thickness of medium of dielectric constant K2 as shown in fig. is given by

CALCULATION OF ELECTRIC FIELD INTENSITY FOR A DISTRIBUTION OF DIRECT AND CONTINUOUS CHARGE

1. Fix origin of the coordinate system where electric field intensity is to be found.

2. Draw the direction of electric field intensity due to the surrounding charges considering one charge at a time.

3. Resolve the electric field intensity in x and y-axis respectively and find ΣEx and ΣEy

4. The resultant intensity is and where θ is the angle between and x-axis.

5. To find the force acting on the charge placed at the origin, the formula F = qE is used.

ENERGY DENSITY

Energy in unit volume of electric field is called energy density and is given by

,

where E = electric field and εo= permittivity of vacuum

ELECTRIC FIELD DUE TO VARIOUS CHARGE DISTRIBUTION

• Electric Field due to an isolated point charge

• A circular ring of radius R with uniformly distributed charge

When x >> R,

[The charge on ring behaves as point charge]

E is max when . Also Emax

• A circular disc of radius R with uniformly distributed charge with surface charge density σ

• An infinite sheet of uniformly distributed charges with surface charge density σ

• A finite length of charge with linear charge density

and

Special case :

For Infinite length of charge,

∴ and 

• Due to a spherical shell of uniformly distributed charges with surface charge density σ

Ein = 0 (x < R)

• Due to a solid non conducting sphere of uniformly distributed charges with charge density ρ

 

 

• Due to a solid non-conducting cylinder with linear charge density λ

Eaxis = 0, 

,

,

In above cases,

 

POINTS TO REMEMBER

1. If the electric lines of force are parallel and equally spaced, the field is uniform.

2. If E0 and E be the electric field intensity at a point due to a point charge or a charge distribution in vacuum and in a medium of dielectric constant K then

E = KE0

3. If E and E' be the electric field intensity at a point in the two media having dielectric constant K and K' then

4. The electric field intensity at a point due to a ring with uniform charge distribution doesn't depend upon the radius of the ring if the distance between the point and the centre of the ring is much greater than the radius of the ring. The ring simply behaves as a point charge.

5. The electric field intensity inside a hollow sphere is zero but has a finite value at the surface and outside it (; x being the distance of the point from the centre of the sphere).

6. The electric field intensity at a point outside a hollow sphere (or spherical shell) does not depend upon the radius of the sphere. It just behaves as a point charge.

7. The electric field intensity at the centre of a non-conducting solid sphere with uniform charge distribution is zero. At other points inside it, the electric field varies directly with the distance from the centre (i.e. E ∝ x; x being the distance of the point from the centre). On the surface, it is constant but varies inversely with the square of the distance from the centre (i.e.). Note that the field doesn't depend on the radius of the sphere for a point outside it. It simply behaves as a point charge.

8. The electric field intensity at a point on the axis of a non-conducting solid cylinder is zero. It varies directly with the distance from the axis inside it (i.e. E ∝ x). On the surface, it is constant and varies inversely with the distance from the axis for a point outside it (i.e. ).

MOTION OF A CHARGED PARTICLE IN AN ELECTRIC FIELD

Let a charged particle of mass m and charge q be placed in a uniform electric field, then electric force on the charge particle is

∴ acceleration, (constant)

• The velocity of the charged particle at time t is,

v = u + at = at = (Particle initially at rest) or

• Distance travelled by particle is

• Kinetic energy gained by particle,

If a charged particle is entering the electric field in a perpendicular direction.

Let and the particle enters the field with speed u along the x-axis.

Acceleration along Y-axis,

The initial component of velocity along the y-axis is zero. Hence the deflection of the particle along y-axis after time t is ;

…… (i)

Distance covered by particle in x-axis,

x = ut …… (ii) ( acceleration ax = 0)

Eliminating t from equation (i) & (ii),

i.e. y ∝ x2.

This shows that the path of a charged particle in a perpendicular field is parabola.

If the width of the region in which the electric field exists be l then

1. the particle will leave the field at a distance from its original path in the direction of field, given by

2. The particle will leave the region in the direction of the tangent drawn to the parabola at the point of escape.

3. The velocity of the particle at the point of escape is given b
   
   

4. The direction of the particle in which it leaves the field is given by

ELECTRIC DIPOLE

Two equal and opposite charges separated by a finite distance constitute an electric dipole. If –q and +q are charges at distance 2l apart, then dipole moment,

Its SI unit is coulomb metre.

Its direction is from –q to +q. It is a vector quantity.

The torque τ on a dipole in uniform electric field as shown in figure is given by,

So τ is maximum, when dipole is ⊥ to field & minimum (=0) when dipole is parallel or antiparallel to field.

If and

Then

The work done in rotating the dipole from equilibrium through an angle dθ is given by

and from θ1 → θ2,

 

If θ1 = 0 i.e., equilibrium position, then

Work done in rotating an electric dipole in uniform electric field from θ1 to θ2 is W = pE (cosθ1 – cosθ2)

Potential energy of an electric dipole in an electric field is,

 

i.e. U = –pE cosθ

where θ is the angle betweenand .

We can also write 

ELECTRIC FIELD DUE TO AN ELECTRIC DIPOLE

• Along the axial line (or end-on position)

and are parallel

when x >> l

• Along equatorial line (or broadside on position)

when x >>l

When and are anti parallel then,

Eax = 2 Eeq

• At any point (from the dipole)

;

Electric field intensity due to a point charge varies inversely as a cube of the distance and in case of quadrupole it varies inversely as the fourth power of distance from the quadrupole.

ELECTRIC FORCE BETWEEN TWO DIPOLES

The electrostatic force between two dipoles of dipole moments p1 and p2 lying at a separation r is

when dipoles are placed coaxially

when dipoles are placed perpendicular to each other.

POINTS TO REMEMBER

1. The dipole moment of a dipole has a direction from the negative charge to the positive charge.

2. If the separation between the charges of the dipole is increased (or decreased) K-times, the dipole moment increases (or decreases) by K-times.

3. The torque experienced by a dipole placed in a uniform electric field has value always lying between zero and pE, where p is the dipole moment and E, the uniform electric field. It varies directly with the separation between the charges of the dipole.

4. The work done in rotating a dipole in a uniform electric field varies from zero (minimum) to 2pE (maximum). Also, it varies directly with the separation between the charges of the dipole.

5. The potential energy of the dipole in a uniform electric field always lies between +pE and –pE.

6. The electric field intensity at a point due to an electric dipole varies inversely with the cube of the distance of the point from its centre if the distance is much greater than the length of the dipole.

7. The electric field at a point due to a small dipole in end-on position is double of its value in broad side-on position,

i.e. EEnd-on = 2EBroad side-on

8. For a small dipole, the electric field tends from infinity at a point very close to the axis of the dipole to zero at a point at infinity.

9. The force between two dipoles increases (or decreases) by K4-times as the distance between them decreases (or increases) by K-times.

10. Time period of a dipole in uniform electric field is

where I = moment of inertia of the dipole about the axis of rotation.

ELECTRIC FLUX

Electric flux is a measure of the number of electric field lines passing through the surface. If surface is not open & encloses some net charge, then net number of lines that go through the surface is proportional to net charge within the surface.

For uniform electric field when the angle between area vector and electric field has the same value throughout the area,

For uniform electric field when the angle between the area vector and electric field is not constant throughout the area

POINTS TO REMEMBER

1. The electric flux is a scalar although it is a product of two vectors and (because it is a scalar product of the two).

2. The electric flux has values lying between –EA and +EA, where E and A are the electric field and the area of cross-section of the surface.

GAUSS'S LAW

It states that, the net electric flux through a closed surface in vacuum is equal to 1/εo times the net charge enclosed within the surface.

i.e.,

where Qin represents the net charge inside the gaussian surface S.

Closed surface of irregular shape which enclosed total charge Qin

In principle, Gauss's law can always be used to calculate the electric field of a system of charges or a continuous distribution of charge. But in practice it is useful only in a limited number of situations, where there is a high degree of symmetry such as spherical, cylindrical etc.

• The net electric flux through any closed surface depends only on the charge inside that surface. In the figures, the net flux through S is q1/εo, the net flux through S’ is (q2 +q3 )/εo and the net flux through S" is zero.

A point charge Q is located outside a closed surface S. In this case note that the number of lines entering the surface equals the number of lines leaving the surface. In other words the net flux through a closed surface is zero, if there is no charge inside.

• The net flux across surface A is zero

i.e.,

because Qin = – q + q = 0

APPLICATIONS OF GAUSS’S LAW

• To determine electric field due to a point charge

The point charge Q is at the centre of the spherical surface shown in figure.

Gaussian surface and is parallel to (direction normal to Gaussian surface) at every point on the Gaussian surface.

so,

• To determine electric field due to a cylindrically symmetric charge distribution

We calculate the electric field at a distance r from a uniform positive line charge of infinite length whose charge per unit length is λ = constant. The flux through the plane surfaces of the Gaussian cylinder is zero, since it is parallel to the plane of the end surface (is perpendicular to ). The total charge inside the Gaussian surface is λl, where λ is linear charge density and l is the length of the cylinder.

Now applying Gauss’s law and noting is parallel to everywhere on cylindrical surface, we find that

POINTS TO REMEMBER

1. The closed imaginary surfaces drawn around a charge are called Gaussian surfaces.

2. If the flux emerging out of a Gaussian surface is zero then it is not necessary that the intensity of the electric field is zero.

3. In the Gauss's law,

is the resultant electric field due to all charges lying inside or outside the Gaussian surface, but Qin is the charge lying only inside the surface.

4. The net flux of the electric field through a closed surface due to all the charges lying inside or outside the surface is equal to the flux due to the charges only enclosed by the surface.

5. The electric flux through any closed surface does not depend on the dimensions of the surface but it depends only on the net charge enclosed by the surface.

Very Short Answer Questions 

Q 1. Does the charge given to a metallic sphere depend on whether it is hollow or solid? Give reason for your answer. [CBSE Delhi 2017]

Ans. No, the charge given to a metallic sphere does not depend on whether it is hollow or solid because the charge only resides on the surface of the conductor. 

Q 2. Two insulated charged copper spheres A and B of identical size have charges qA and –3qA respectively. When they are brought in contact with each other and then separated, what are the new charges on them? [CBSE (F) 2011]

Ans. Charge on each copper sphere = qA – 3qA/2 = – qA

Q 3. What is the electric flux through a cube of 1 cm which encloses an electric dipole? [CBSE Delhi 2015]

Ans. The net electric flux will be zero. It is so because:

(i) Electric Flux is independent of the shape and size of the cube.

(ii) Net charge of the electric dipole is zero. 

Q 4. What orientation of an electric dipole in a uniform electric field corresponds to its (i)stable and (ii)unstable equilibrium? [CBSE Delhi 2010] [HOTS] 

Ans. (i) In stable equilibrium the dipole moment is parallel to the direction of the electric field (i.e., θ = 0).

(ii) In unstable equilibrium PE is maximum, so θ = π, i.e; the dipole moment is antiparallel to the electric field.

Q 5. Define electric field strength. Is it a vector or a scalar quantity? 

Ans. The electric field strength at a point in an electric field is defined as the electrostatic force acting on a unit positive charge when placed at that point and its direction is along the direction of electrostatic force. Electric field strength is a vector quantity.

Q 6. What kind of charge is produced while rubbing a glass rod and silk together?

Ans. While rubbing glass rod and silk together, they produce opposite charges. While the glass rod has a negative charge, the silk will have a positive charge.

Q 7.What is the name of the experiment that establishes the quantum nature of electric charge?

Ans. The experiment that establishes the quantum nature of electric charge is called – Millikan’s Oil Drop Experiment.

Q 8.A body can have a charge 8.0 x 10-20 C. Explain if the statement is true or false with logic.

Ans. This statement is false, a body can not have a charge 8.0 x 10-20 C, as it is less than the charges of electrons and protons, which determines the core charge of a body. Hence, the minimum charge a body can have is- 1.6 x 10-19.

Q 9.  Is Newton’s third law maintained by Coulomb's Law?

Ans. Coulomb Law maintains Newton’s Third Law of Motion. It states “the force experienced by two charges are equal in magnitude  but mutually opposite in direction”.

Q 10. Two equal balls having equal positive charge ‘q’ coulombs are suspended by two insulating strings of equal length. What would be the effect on the force when a plastic sheet is inserted between the two? [CBSE AI 2014]

Ans. The force will decrease upon inserting a plastic sheet. 

Reason: Force between two charges each ‘q’ in vacuum is 

F0=9x109. q2/r2

On inserting a plastic sheet (a dielectric K > 1) 

Then F=9x109. q2/Kr2.

  i.e., Force F=F0  /K

Part One : Electrostatics

What is Electrostatics

In your childhood, have you played the plastic chair and towel game? If you haven’t played yet, then let's try this interesting game. For this game, you require two to three people, one plastic chair and a towel. First of all, one person should sit on the chair with both feet above the ground (no body part should be in contact with the ground or any other thing except the chair) and then one person takes a towel and hits the back of the chair for 30 seconds. When someone will touch the person who is sitting on the chair, he or she will get a very mild electric shock. Now if you are wondering how can this happen, then the concepts of electrostatics will help you to understand this phenomenon. Electrostatics constitutes of two words “Electro” means electron or charge and “Static” means at rest. So in this plastic chair and towel game, due to the beating of the towel, the charges are generated and we get shocked due to these static charges. The similar phenomenon is when we comb our hair on a dry day and bring the comb close to tiny pieces of paper, we note that they are swiftly attracted by the comb.

 Electrostatics is a branch of physics that studies electric charges at rest or static electricity.

Electrostatic phenomena arise from the forces that electric charges exert on each other. There are many examples of electrostatic phenomena: The attraction of the plastic wrap to your hand after you remove it from a package. The attraction of paper to a charged scale. The apparently spontaneous explosion of grain silos. The damage of electronic components during manufacturing.

Part Two : Electric Charge

Electric charge

Electric charge is a fundamental property of matter and the foundation for electricity or in simpler words we can say that, Electric charge is the physical property of matter that causes it to experience a force when placed in an electromagnetic field.

      

Electrons are known to have a negative charge while protons have a positive charge.

What is Electric Charge

1.  Electric Charge is the property of subatomic particles that causes it to experience a force when placed in an electric and magnetic field. 
2.  Electric charges are of two types: Positive and Negative, commonly carried by charge carriers protons and electrons.

3.   Like charges repel each other and unlike charges attract each other.

       Examples of the types of charges are subatomic particles or the particles of matter:
            Protons are positively charged.
            Electrons are negatively charged.
            Neutrons have zero charge.

4. Electric charge is a scalar quantity.

5. Electric charges are additive in nature.

6. Electric charges are quantized.

7. Electric charges are conserved.

These last three properties of charge are called characteristics of Electric charge.

• Additivity of Electric Charge

            For a system containing n particles, the total charge of the system can be written as,

• Conservation of Electric Charge

              In an isolated system, Electric charge is conserved, which means the net electric charge of the                                system is constant. The algebraic sum of the fundamental charges in any isolated system remains 

              the same.

• Quantization of Electric Charge

             According to the principle of quantization of electric charge, all the free charges are integral multiples of a basic predefined unit which we denote by e. Thus, the charge possessed by a system can be given as,

               Where n is an integer (zero, a positive or a negative number) and e is the basic unit of charge, that is, the charge carried by an electron or a proton. The value of e is 1.6 × 10-19C.

       

 

Like charges repel each other and unlike charges attract each other.

Charging Or Electrification

The process of supplying the electric charge to an object or transferring the electric charge from an object is called charging.

An uncharged object can be charged in three different ways as follows:

• Charging by friction  
• Charging by conduction
• Charging by induction

Charging by Friction

When two objects are rubbed against each other, charge transfer takes place. One of the objects loses electrons while the other object gains electrons. The object that loses electrons becomes positively charged and the object that gains electrons becomes negatively charged. Both the objects get charged due to friction and this method of charging is commonly known as electrification by friction.

Charging by Conduction

The method of charging an uncharged object by bringing it close to a charged object is known as charging by conduction. The charged conductor has an unequal number of protons and electrons, hence when an uncharged conductor is brought near it, it discharges electrons to stabilize itself.

Charging by Induction

The process of charging an uncharged conductor by bringing it near a charged conductor without any physical contact is known as charging by induction.

  Electric Force  

     Electric Force is the repulsive or attractive interaction between any two charged bodies.  

    In Electrostatics, these are called Coulomb forces too. 

The basic difference between gravitational force and electric force is that the former one only attracts while the latter one attracts only if the charges are of opposite nature (positive and negative) and a repulsive force acts if the charges have same nature. 

Part Three : Coulomb's Law.

 Formula for Coulomb’s Law of electrostatics

+Q and +q charges are at r distance

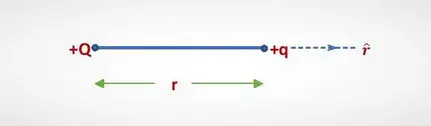
Let two point charges +Q and +q are placed at a distance of separation r. Then according to Coulomb’s law, the electric force (F) acting between the charges is,

$\small {\color{Blue} F\propto Qq}$ and,

 $\small {\color{Blue}F\propto \frac{1}{r^{2}}}$

$\small {\color{Blue} F\propto \frac{Qq}{r^{2}}}$Then we get,    

$F\propto \frac{Qq}{r^2}$

or, ${\color{Blue}F=\frac{kQq}{r^{2}}}$ ………………….. (1)

Where, k is the Coulomb’s constant. It varies medium to medium.

In CGS unit, the value of k is 1 and in SI unit, k has an expression as ${\color{Blue} k=\frac{1}{4\pi \epsilon _{0}}}$ in air medium or free space which have the value of 9×109. Here, $\small {\color{Blue} \epsilon _{0}}$ is the permittivity of free space.

So, the equation for Coulomb’s law in air medium is,

         $\small {\color{Blue} F=\frac{1}{4\pi \epsilon _{0}}\frac{Qq}{r^{2}}}$ ……(2) in SI unit.

and, $\small {\color{Blue} F=\frac{Qq}{r^{2}}}$ ………………. (3) in CGS unit.

For the other medium, one should use the permittivity of that medium in place of the permittivity of free space.

Vector form of Coulomb’s Law 

In SI system, the magnitude of electrostatic force is given by the equation-(2). Now, the force is repulsive for two positive charges +Q and +q. So, the force on q will act along the outward direction from q. We denote the unit vector by ${\color{Blue} \widehat{r}}$ along the outward direction from q.

Then the vector form of Coulomb’s law is, $\small {\color{Blue} \overrightarrow{F}=\frac{1}{4\pi \epsilon _{0}}\frac{Qq}{r^{2}}\widehat{r}}$ …….. (4)

Again, the position vector is, $\small {\color{Blue} \overrightarrow{r}=r\widehat{r}}$. Then, $\small {\color{Blue} \widehat{r}=\frac{\overrightarrow{r}}{r}}$……….. (5)

Now, from equation-(4) and equation-(5) one can get, $\small {\color{Blue} \overrightarrow{F}=\frac{1}{4\pi \epsilon _{0}}\frac{Qq}{r^{3}}\overrightarrow{r}}$ ……… (6).

Equation-(6) is the another form of the vector form of Coulomb’s law.

Derivation of Coulomb’s Law from Gauss’s Law

The equation of Coulomb law can be derived from the Gauss law of electrostatics.

According to the Gauss’s law of electrostatics, the electric flux passing through a spherical surface of charge Q and radius r is, $\small {\color{Blue} \int \overrightarrow{E}.\overrightarrow{dS}=\frac{Q}{\epsilon _{0}}}$.

Then the electric field on the surface of the sphere due the Q charge is, $\small {\color{Blue} E=\frac{Q}{4\pi \epsilon _{0}r^{2}}}$.

If we place another charge q on the surface of the sphere, the electrostatic force on the charge q will be, F = qE.

Now, putting the equation of E in F = qE we get, $\small {\color{Blue} F=\frac{Qq}{4\pi \epsilon _{0}r^{2}}}$.

This is nothing but the Coulomb’s force equation. Hence, Coulomb’s Law is derived from the Gauss’s law.

Application of Coulomb law

The only application of Coulomb’s equation is to find the electric force between the static electric charges. One can find the value of electric field at any point by observing the Coulomb’s force at that point.

Limitations of Coulomb’s law

Here are some limitations of Coulomb law –

1. Coulomb’s law is applicable only for the point charges. To apply this law for a large body its total charge is assumed to be located at a single point (at Centre of charge) so that it can be treated as a point charge.
2. This law is valid for the charges at rest with respect to the observer.
3. Coulomb’s law is valid when the distance between the charges is greater than the nuclear distance or nuclear diameter (10-15m).

Coulomb’s Law 

Coulomb’s law is an experimental law that quantifies the amount of force that exists between two stationary electrically charged particles which is also conventionally known as the electrostatic force or Coulomb’s force.  

Coulomb’s law states that:

"The electrostatic force of attraction or repulsion between two point charges is directly proportional to the scalar multiplication of the two charges and inversely proportional to the square of the distance between them."

Scalar Form of Coulomb's Law

Vector Form of Coulomb's Law

The vector form of Coulomb's law gives both magnitude and direction of an electric force.

There are two cases depending on the nature of the charges interacting.

Case-1 : When charges are alike.

(Both are positive or both are negative.)

 q₁ >0, q₂ >0 or q₁ <0, q₂<0

Forces are repulsive in nature.

Case-2 : When charges are unlike.(Opposite charges)

q₁ >0, q₂ <0 or q₁ <0, q₂ >0,

Forces are attractive in nature.

 

Electric Force exerted by one point charge on another act along the line between the charges. It varies inversely as the square of the distance separating the charges and is proportional to the product of charges.